- Enseignant: Ralf Gerkmann
- Enseignant: Simon Gritschacher
- Enseignant: Enya Hsiao
- Enseignant: Paula Pilatus
- Enseignant: Jonas Stelzig
- Enseignant: Christian Fries
- Enseignant: Andrea Mazzon
- Enseignant: Andreas Rosenschon
- Enseignant: Simon Weinzierl
- Enseignant: Andreas Rosenschon
- Enseignant: Simon Weinzierl
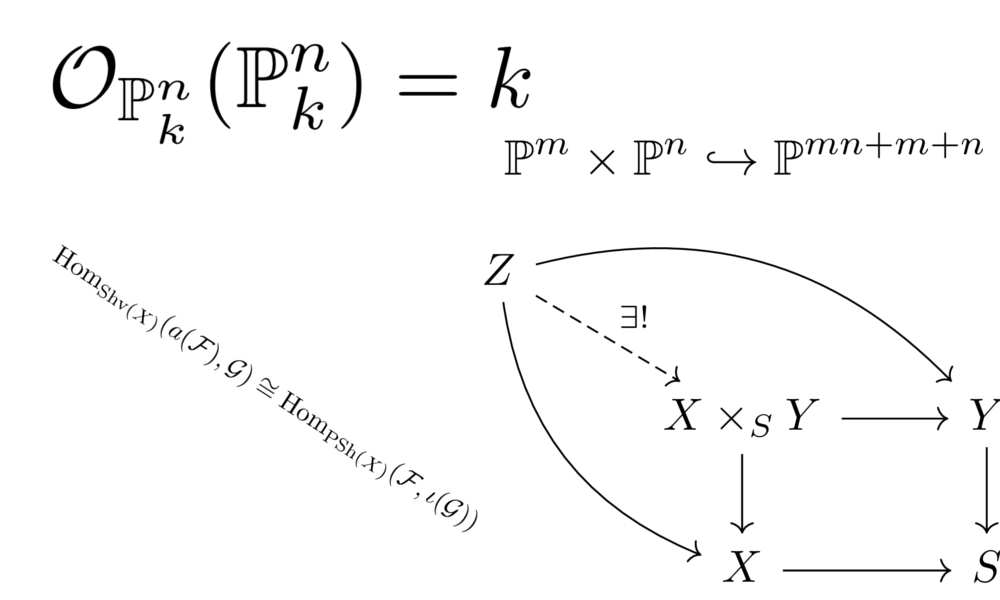
In diesem Modul wird in die Algebraische Geometrie, insbesondere in die Theorie der algebraischen Varietäten und/oder Schemata und ihrer Morphismen eingeführt. Es werden affine und projektive Varietäten bzw. Schemata studiert und grundlegende Eigenschaften untersucht, insbesondere Dimension, Morphismen und birationale Abbildungen.
- Enseignant: Andreas Rosenschon
- Enseignant: Simon Weinzierl
- Enseignant: Ulrich Bauer
- Enseignant: Thomas Vogel
- Enseignant: Lukas Böke
- Enseignant: Thomas Vogel
- Enseignant: Bernhard Leeb
- Enseignant: Leopold Zoller
- Enseignant: Lukas Gonon
- Enseignant: Niklas Walter
- Enseignant: Alejandro Caicedo
- Enseignant: Markus Heydenreich
The lecture provides an introduction to stochastic calculus with an emphasis on the mathematical concepts that are later used in the mathematical modeling of financial markets.
In the first part of the
lecture course the theory of stochastic integration with respect to
Brownian motion and Ito processes is developed. Important results such
as Girsanov's theorem and the martingale representation theorem are also
covered. The first part concludes with a chapter on the existence and
uniqueness of strong and weak solutions of stochastic differential
equations.
The second part of the lecture course gives an introduction to the arbitrage theory of financial markets in continuous time driven by Brownian motion. Key concepts are the absence of arbitrage, market completeness, and the risk neutral pricing and hedging of contingent claims. Particular attention will be given to the the Black-Scholes model and the famous Black-Scholes formula for pricing call and put options.
If you wish to participate in the
course, please sign up as soon as possible by sending an e-mail from
your LMU e-mail address to Annika Steibel (steibel@math.lmu.de).
- Enseignant: Thilo Meyer-Brandis
- Enseignant: Annika Steibel
- Enseignant: Konstantinos Panagiotou
- Enseignant: Simon Reisser
- Enseignant: Ari-Pekka Perkkiö
- Enseignant: Simon Reisser
- Enseignant: Konstantinos Panagiotou
- Enseignant: Pascal Stucky